Conversion of twisted light to twisted excitons using carbon nanotubes

Abstract

Carbon nanotubes are explored as a means of coherently converting the orbital angular momentum of light to an excitonic form that is more amenable to quantum information processing. An analytical analysis, based on dynamical conductivity, is used to show that orbital angular momentum is conserved, modulo N, for a carbon nanotube illuminated by radially polarized, twisted light. This result is numerically demonstrated using real-time time-dependent density functional theory which captures the absorption of twisted light and the subsequent transfer of twisted excitons. The results suggest that carbon nanotubes are promising candidates for constructing optoelectronic circuits in which quantum information is more readily processed while manifested in excitonic form.

Introduction

Centrosymmetric molecules can host twisted excitons carrying orbital angular momentum (OAM)¹, and this can be employed to mediate algebraic operations involving twisted light². A chain of these molecules constitutes a conduit for OAM transfer in the form of twisted exciton wave packets with controllable linear momentum^3,4. The interconversion and manipulation of optical and excitonic manifestations of OAM represents a rich field for quantum information processing⁵. While optical methodologies are already well-established for encoding and transmitting data as OAM^{6,7,8,9,10,11}, a distinct advantage of excitons is that they can be readily combined and manipulated for quantum information processing¹. A chain of molecules is therefore qualitatively different than simply directing twisted light through optical fibers¹². The experimental realization of twisted excitons, however, is challenging, because it requires rigidly connected molecules with low reorganization energy.

Carbon nanotubes (CNTs) offer a means transferring the angular momentum of light to excitonic form, processing it, and then converting it back into an optical form. This is because they are structurally rigid and have the requisite C_N symmetry¹³. The Bloch wave functions, therefore, can be constructed using the rotational operator with the OAM as eigenvalue. The fact that the electrons carry OAM has been confirmed theoretically¹⁴ and experimentally¹⁵. Ultra-long CNTs have been experimentally fabricated^16,17 to lengths of as much as half a meter¹⁸. CNTs with carbon purity above 99.98% can be synthesized very efficiently¹⁹ and 92% yield has been reported for growth of CNTs with specific chirality²⁰. Combined with tunable band gaps, CNTs are promising candidates for optoelectronic applications as in, for example, solar cells^{21,22,23,24,25,26,27,28,29}. Recently, CNTs are demonstrated to be high-purity and high-efficiency single-photon sources, which makes CNTs promising candidates for on-chip quantum light sources for quantum information³⁰. Although a lot is known about the optoelectronic properties of CNTs³¹ and their exciton dynamics^32,33,34,35, the interaction with twisted light is essentially unexplored³⁶.

Here we computationally demonstrate that OAM is conserved when CNTs convert twisted light into twisted excitons. We first establish the optical selection rules by deriving the dynamical conductivity based on the helical and rotational symmetries. Then real-time time-dependent density functional theory (RT-TDDFT) is employed to directly simulate the transfer of OAM from twisted light to twisted excitons³⁷. RT-TDDFT includes the multi-level and multi-electron effects omitted by the tight-binding model. We show that CNTs have effectively the same structure as a molecular chain except that there is a constant helical misorientation between neighboring sites as illustrated in Fig. 1a. CNTs are readily available with a wide range of electronic band structures. They are also rigid and have low reorganization energy so that exciton phonon coupling is minimized, helping to support coherence³⁸. Although the extended states of CNTs make this intuitively reasonable, a reorganization energy of tens of meV has been reported in a recent experiment³⁹. The reorganization energy estimated by the peak width of photoluminescence spectra ranges from tens of meV⁴⁰ to as low as 1 meV⁴¹. This is comparable to the most rigid porphyrins^42,43. CNTs therefore offer an ideal setting for the realization of twisted exciton circuitry.

Fig. 1: CNTs and OAM.

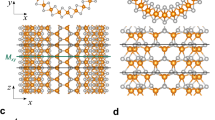
a CNTs have the same structure as a chain of coaxial parallel molecules with helical misorientation. A chain of centrosymmetric molecules exhibits a structural helicity in which each molecule is misoriented relative to its neighbors. Exciton hopping can still occur between nearest-neighbor arms of the same color. b Molecule with C₃ symmetry. c Clockwise 2π phase variation for OAM = 1 and d counterclockwise 4π phase variation for OAM = −2. Applied to the molecule in b, by taking the phases of c and d at the positions of the arms, twisted light with e OAM = 1 and f OAM = −2 leads to the same phase difference between neighboring arms modulo 2π.

The central concept can be elucidated using a tight-binding model with coupling between the p_z orbitals of neighboring carbon atoms. The resulting band structure and eigenstates can then be expressed in terms of crystal momentum due to translational symmetry along the principle axis of the CNT⁴⁴. However, the required computational time can be dramatically reduced by exploiting helical and rotational symmetries instead⁴⁵. This approach also is advantageous in that the OAM is related to irreducible representations of SO(2) for continuous space and C_N for discrete space. Each eigenstate can be identified with a helical quantum number, κ, for the twist of subsequent structural units along the axis and a rotational quantum number, q, due to the rotational symmetry of structural strands of which the tube is composed. Phonon effects are disregarded under the reasonable assumption that reorganization energy is so low for the extended states of this system exciton wave packet coherence is maintained during the information processing. This can be elucidated by considering a dimer consisting of the first two sites in Fig. 1a^38,46, for which the decoherence rate R can be analytically calculated as the sum of a pure dephasing rate R_d and a relaxation rate R_r:

$$R={R}_{d}+{R}_{r}$$

$${R}_{d}=\delta {\varepsilon }^{2}S(0)/(2{b}^{2})$$

(1)

$${R}_{r}\approx 4{J}^{2}S(b)/(2{b}^{2}).$$

(2)

Here b² = δε² + 4J² with δε and J being the onsite energy difference and the coupling between these two sites, respectively. The thermally weighted spectral density is

$$S(\omega )=2\pi \coth [\hslash \omega /(2{k}_{B}T)]\mathop{\sum}\limits_{i}{\omega }_{i}{d}_{i}^{2}\delta (\omega -{\omega }_{i})$$

(3)

with d_i, ω_i and K_B being the strength of electron-phonon coupling, the frequency of the phonon mode, and Boltzmann’s constant, respectively. The reorganization energy is $\lambda ={\sum }_{i}\hslash {\omega }_{i}{d}_{i}^{2}\equiv {\sum }_{i}\lambda ({\omega }_{i})$. Since all sites in a given CNT have same onsite energy, we have R_d = 0 and b = 2∣J∣ = 2∣V_ppπ∣ where V_ppπ = −2.77 eV⁴⁷ is the coupling between nearest p_z orbitals in CNTs. Thus R = πλ(b)/ℏ is much less than πλ/ℏ = 1/21fs⁻¹ and the coherence time 1/R is much larger than 21 fs. This result is based on the estimate that λ = 10 meV. Both the coherence time and the exciton life time, which is estimated to be in the order of 10 ps⁴¹, are much larger than the exciton transfer time in a 4 nm (3,3) CNT (see Fig. 7). Then a momentum conservation is associated with the photo-excitation of an electron from a valence state with quantum numbers κ and q to a conduction state with quantum numbers $\kappa ^{\prime}$ and $q^{\prime}$. Specifically, $q^{\prime} -q$ and $\kappa ^{\prime} -\kappa$ must be equal to the photonic angular momentum (PAM) and helical momentum of the twisted light, respectively.

The OAM conservation between excitons and photons can be studied within the context of band structure. The OAM of the exciton (XAM) is the total OAM of all occupied Bloch states and is also the sum of the OAM of all electrons in conduction bands (EAM) and all holes in valence bands (HAM). The fact that the ground state has zero OAM indicates that the EAM associated with an excited electron is the negative of the total EAM from all other electrons. This means that the electron and hole in the same Bloch state are of opposite OAM. It is shown that OAM is conserved during an optical excitation, i.e., PAM = XAM = EAM + HAM. In the absence of meaningful phonon effects, a twisted exciton wave packet transfers down the CNT with the same OAM as was absorbed from twisted light.

Results

Tight-binding analysis

A CNT can be created by rolling up graphene along a lattice vector $\overrightarrow{R}={n}_{1}{\overrightarrow{e}}_{1}+{n}_{2}{\overrightarrow{e}}_{2}$ with the resulting structure characterized by the pair of integers (n₁, n₂). Here ${\overrightarrow{e}}_{2}$ and ${\overrightarrow{e}}_{2}$ are the primitive lattice vectors (Fig. 2). A band gap is present provided⁴⁴

$${n}_{1}-{n}_{2}=3l\pm 1,\quad l\in {\mathbb{Z}}.$$

(4)

Examination of the resulting helical and rotational symmetries⁴⁵ reveals that each CNT can be viewed as an M-site system, with N arms at each site, in which each arm consists of two inequivalent carbon atoms denoted as A and B. For the (5, 10) CNT of Fig. 2, for instance, there are five arms at each site which are created by rotational operations of the first arm, and these five arms give rise to the entire CNT by M − 1 subsequent helical operations. Figure 3 shows the spiral formed by following one arm along the different sites. The primitive lattice vector along the helical direction is given by $\overrightarrow{H}={p}_{1}{\overrightarrow{e}}_{1}+{p}_{2}{\overrightarrow{e}}_{2}$ with p₁ ≤ p₂ being the smallest nonnegative integers fulfilling p₁n₂ − p₂n₁ = N.

Fig. 2: Decomposition of the (5,10) CNT into a spiral of 5-arm sites.

The CNT can be viewed as a piece of graphene rolled up along the lattice vector $\overrightarrow{R}=5{\overrightarrow{e}}_{1}+10{\overrightarrow{e}}_{2}$ (${\overrightarrow{e}}_{1}$ and ${\overrightarrow{e}}_{2}$ being the primitive lattice vectors). Each arm consists of two inequivalent carbon atoms, denoted as A and B. The 5 arms are highlighted by different colors. $\overrightarrow{H}$ is the primitive lattice vector along the spiral. The black dashed lines indicate different sites.

Fig. 3: Geometry.

Spiral of arm 1 (red color) with angle θ between sites 1 and 2. The phase of the twisted light is zero along the z-direction (black line).

Since our goal is not to generate quantitatively precise band structures, a π-band approximation is applied to demonstrate the key idea that CNTs can serve as conduits for OAM transport and thus as building blocks for optoelectronic circuits. The symmetry group generated by the helical operation is isomorphic to the one-dimensional translation group, so that Bloch’s theorem can be readily generalized⁴⁷. Moreover, because the helical and rotational operators commute, we obtain the following symmetry-adapted, generalized Bloch sums⁴⁵:

$$|{{{\Phi }}}_{\kappa ,q}^{\alpha }\rangle =\frac{1}{\sqrt{MN}}\mathop{\sum }\limits_{m=1}^{M}\mathop{\sum }\limits_{n=1}^{N}{\varepsilon }_{M}^{(m-1)\kappa }{\varepsilon }_{N}^{(n-1)q}|{\phi }_{m,n}^{\alpha }\rangle .$$

(5)

Here ε_M = e^i2π/M and ε_N = e^i2π/N. Each Bloch sum is given by three parameters: α, κ, and q. While α is A or B (inequivalent carbon atoms), κ and q are the quantum numbers associated with the helical and rotational operators, respectively, taking on integer values within the domains $(-\frac{M}{2},\frac{M}{2}]$ and $(-\frac{N}{2},\frac{N}{2}]$. M is the site number after M − 1 helical operations and N is the arm number after N − 1 rotational operations. Moreover, $|{\phi }_{m,n}^{\alpha }\rangle$ is the orbital of the α-type carbon atom in arm n at site m.

The matrix elements between Bloch sums with different κ and q are zero. Thus, the wave functions are linear combinations of basis states,

$$|{{{\Psi }}}_{\kappa ,q}\rangle ={c}_{\kappa ,q}^{A}|{{{\Phi }}}_{\kappa ,q}^{A}\rangle +{c}_{\kappa ,q}^{B}|{{{\Phi }}}_{\kappa ,q}^{B}\rangle ,$$

(6)

with constants ${c}_{\kappa ,q}^{A}$ and ${c}_{\kappa ,q}^{B}$. Inserting this into the Schrödinger equation gives a 2 × 2 eigenvalue problem with energies, ± ∣H_AB∣, that are functions of the quantum numbers κ and q:

$$\begin{array}{ll}{H}_{AB}\equiv \langle {{{\Phi }}}_{\kappa ,q}^{A}|{\hat{H}}_{0}|{{{\Phi }}}_{\kappa ,q}^{B}\rangle \\\qquad\, ={V}_{pp\pi }\mathop{\sum}\limits_{\langle 1,1;i,j\rangle }{\varepsilon }_{M}^{(i-1)\kappa }{\varepsilon }_{N}^{(j-1)q}.\end{array}$$

(7)

Here V_ppπ is the coupling strength between nearest neighbors (with a value of −2.77 eV⁴⁷), 〈1, 1; i, j〉 denotes the nearest neighbors of atom A in arm 1 at site 1, and ${\hat{H}}_{0}$ is the one-electron Hamiltonian of the CNT. This allows the band structure (which is a function of κ and q) to be immediately constructed. The eigenstates of ${\hat{H}}_{0}$ are the Bloch wave functions $\left|s,q,\kappa \right\rangle$,

$${\hat{H}}_{0}\left|s,q,\kappa \right\rangle ={\varepsilon }_{s,q,\kappa }\left|s,q,\kappa \right\rangle ,$$

(8)

where s = +/− refers to conduction/valence states.

Turning to the interaction of CNTs with twisted light, an ideal Bessel mode can be written as^48,49

$$E(\rho ,\phi ,z,t)={J}_{l}({k}_{\rho },\rho ){{{{\rm{e}}}}}^{{{{\rm{i}}}}l\phi }{{{{\rm{e}}}}}^{{{{\rm{i}}}}{k}_{z}z}{{{{\rm{e}}}}}^{-{{{\rm{i}}}}\omega t},$$

(9)

where J_l denotes the l-th order Bessel function of the first kind, (ρ, ϕ, z) the polar coordinates, k_z and k_ρ the wave numbers, and ω the frequency. For cylindrically polarized twisted light^50,51 with polarization singularity on the main axis of the CNT, the rotational center of the SO(2) symmetry (twisted light) coincides with that of the C_N symmetry (CNT). Thus, the combined system (twisted light and CNT) and, particularly, the Hamiltonian of the light-matter interaction possess rotational symmetry, i.e., the OAM may be conserved. We adopt radially polarized twisted light, i.e.,

$$\overrightarrow{E}={\overrightarrow{e}}_{\rho }{E}_{0}\sin (l\phi +{k}_{z}z-\omega t),$$

(10)

with ${\overrightarrow{e}}_{\rho }$ being the radial unit vector and E₀ the magnitude of the electric field on the surface of the CNT.

Note that e^ilϕ with ϕ ∈ (0, 2π] are the irreducible representations of the rotation group of continuous two-dimensional space, SO(2), where l can be any integer. When twisted light is applied to a CNT the rotation group becomes C_N with the defining representation ${{{{\rm{e}}}}}^{{{{\rm{i}}}}\frac{(n-1)2\pi }{N}}$. Since the group is abelian, each element (n = 1, ⋯ , N) forms an equivalence class and the number of equivalence classes equals the number of irreducible representations (which must be orthogonal to each other). The dimensions d_r of the irreducible representations must satisfy $\mathop{\sum }\nolimits_{r = 1}^{N}{d}_{r}^{2}=N$, implying d_r = 1. The identity and defining representation are irreducible representations. As $\mathop{\sum }\nolimits_{n-1}^{N}{{{{\rm{e}}}}}^{{{{\rm{i}}}}(q-q^{\prime} )\frac{n-1}{N}2\pi }={\delta }_{q,q^{\prime} }$, the irreducible representations have the form ${{{{\rm{e}}}}}^{{{{\rm{i}}}}q\frac{n-1}{N}2\pi }$, with q to be determined. The character orthogonality requires ${\sum }_{q}{{{{\rm{e}}}}}^{{{{\rm{i}}}}(n-n^{\prime} )\frac{q}{N}2\pi }={\delta }_{n,n^{\prime} }$, which is equivalent to choosing q as integers in the interval $(-\frac{N}{2},\frac{N}{2}]$. Therefore, C_N has the irreducible representations ${{{{\rm{e}}}}}^{{{{\rm{i}}}}q\frac{(n-1)2\pi }{N}}$, n = 1, ⋯ , N and q is an integer in the interval $(-\frac{N}{2},\frac{N}{2}]$. Thus, l is reduced to q modulo N. For example, l = 1 and −2 are equivalent in the case of C₃ symmetry, as both are reduced to q = 1, see Fig. 1b–f. This implies

$$\overrightarrow{E}={\overrightarrow{e}}_{\rho }{E}_{0}\sin \left(\frac{q(n-1)2\pi }{N}+\frac{{\kappa }_{0}(m-1)2\pi }{M}-\omega t\right),$$

(11)

where κ₀ = Mlθ/2π with $\theta =2\pi (\overrightarrow{R}\cdot \overrightarrow{H})/| \overrightarrow{R}{| }^{2}$, see Fig. 3. Note that k_z in Eq. (10) is set to zero based on the dipole approximation, i.e., the wave length of the twisted light is assumed to be much larger than the size of the CNT. The helical momentum κ₀ is nonzero, as the phase of the twisted light is zero along the z-direction, see Fig. 3. Figure 4a shows the values of θ for CNTs with C_N symmetry (N > 2). For N ≤ 2 only excitons with XAM = 0 can be conducted, which is not of our interest. According to Fig. 4b, θ decreases when n₂ increases for both the zigzag and armchair CNTs.

Fig. 4: Evaluation of θ.

Values of θ for (a) (n₁, n₂) CNTs with rotational symmetry C_N (N > 2) and (b) zigzag (red) and armchair (green) CNTs.

The Hamiltonian of the light-matter interaction is

$$\begin{array}{lll}{\hat{H}}_{int}=-e\overrightarrow{r}\cdot {\overrightarrow{e}}_{\rho }{E}_{0}\sin (\psi -\omega t)\\ \qquad\,=\,{D}_{1}{E}_{0}\sin (\omega t)-{D}_{2}{E}_{0}\cos (\omega t),\end{array}$$

(12)

where we define $\psi =\frac{q(n-1)2\pi }{N}+\frac{{\kappa }_{0}(m-1)2\pi }{M}$ as the angle between ${\overrightarrow{e}}_{x}$ and ${\overrightarrow{e}}_{\rho }$, ${D}_{1}\equiv e{r}_{0}\cos \psi$, and ${D}_{2}\equiv e{r}_{0}\sin \psi$ (r₀ being the radius of the CNT). The Cartesian coordinate system is chosen such that D₁ and D₂ refer to the x and y-directions, respectively. The electron density propagates according to the quantum Liouville equation $\frac{\partial \hat{\rho (t)}}{\partial t}=\frac{1}{{{{\rm{i}}}}\hslash }[{{\hat H}(t)},{\hat \rho (t)}]$, where ${\hat H(t)}={\hat{H}}_{0}+{\hat{H}}_{int}$. Considering ${\hat{H}}_{int}$ as perturbation, separating ${\hat \rho (t)}$ into equilibrium and perturbation parts, ${\hat \rho (t)}={\hat{\rho }}_{0}+{{\Delta }}{\hat \rho (t)}$, and omitting the second order term, we obtain

$$\begin{array}{lll}\frac{\partial {{\Delta }}{\hat \rho (t)}}{\partial t}&=\frac{1}{{{{\rm{i}}}}\hslash }\left\{[{\hat{H}}_{0},{{\Delta }}{\hat \rho (t)}]\right.\\ &\left.+\,{E}_{0}\sin (\omega t)[{D}_{1},{\hat{\rho }}_{0}]-{E}_{0}\cos (\omega t)[{D}_{2},{\hat{\rho }}_{0}]\right\}.\end{array}$$

(13)

This equation is solved by

$$\begin{array}{lll}{{\Delta }}{\hat \rho (t)}&=&\frac{1}{{{{\rm{i}}}}\hslash }\int\nolimits_{-\infty }^{t}[{\hat{D}}_{1}(t^{\prime} -t),{\hat{\rho }}_{0}]{E}_{0}\sin (\omega t^{\prime} )dt^{\prime} \\ &&-\,\frac{1}{{{{\rm{i}}}}\hslash }\int\nolimits_{-\infty }^{t}[{\hat{D}}_{2}(t^{\prime} -t),{\hat{\rho }}_{0}]{E}_{0}\cos (\omega t^{\prime} )dt^{\prime} ,\end{array}$$

(14)

where ${\hat{D}}_{i}(t)={\hat{U}}_{0}^{{\dagger} }(t){D}_{i}{\hat{U}}_{0}(t)$ with ${\hat{U}}_{0}(t)={{{{\rm{e}}}}}^{-\frac{{{{\rm{i}}}}}{\hslash }{\hat{H}}_{0}t}$. The current then is given by

$$\begin{array}{lll}\overrightarrow{j}(t)&=&\frac{1}{{{{\rm{i}}}}\hslash }\int\nolimits_{-\infty }^{t}{{{\rm{Tr}}}}\left\{[{\hat{D}}_{1}(t^{\prime} -t),{\hat{\rho }}_{0}]{\hat{\overrightarrow{j}}}(0)\right\}{E}_{0}\sin (\omega t^{\prime} )dt^{\prime} \\ &&-\frac{1}{{{{\rm{i}}}}\hslash }\int\nolimits_{-\infty }^{t}{{{\rm{Tr}}}}\left\{[{\hat{D}}_{2}(t^{\prime} -t),{\hat{\rho }}_{0}]{\hat{\overrightarrow{j}}}(0)\right\}{E}_{0}\cos (\omega t^{\prime} )dt^{\prime} ,\end{array}$$

(15)

with

$$\begin{array}{lll}{\hat{\overrightarrow{j}}}(t)={\hat{j}}_{1}(t){\overrightarrow{e}}_{x}+{\hat{j}}_{2}(t){\overrightarrow{e}}_{y}+{\hat{j}}_{3}(t){\overrightarrow{e}}_{z}\\\qquad =\frac{e}{\pi {r}_{0}^{2}M}\frac{{{{\rm{i}}}}}{\hslash }{\hat{U}}_{0}^{{\dagger} }(t)[{\hat{H}}_{0},{\hat{\overrightarrow{r}}}]{\hat{U}}_{0}(t).\end{array}$$

(16)

From Eq. (15), we have

$$\begin{array}{lll}{j}_{i}(t)=&-&\int\nolimits_{-\infty }^{\infty }{{\Theta }}(t-t^{\prime} ){\alpha }_{i,1}(t-t^{\prime} ){E}_{0}\sin (\omega t^{\prime} ){{{\rm{d}}}}t^{\prime} \\ &+&\int\nolimits_{-\infty }^{\infty }{{\Theta }}(t-t^{\prime} ){\alpha }_{i,2}(t-t^{\prime} ){E}_{0}\cos (\omega t^{\prime} ){{{\rm{d}}}}t^{\prime} ,\end{array}$$

(17)

where Θ(t) is the step function and

$$\begin{array}{lll}{\alpha }_{i,i^{\prime} }(t)&\equiv &-\frac{1}{{{{\rm{i}}}}\hslash }{{{\rm{Tr}}}}\left\{[{\hat{D}}_{i^{\prime} }(t),{\hat{\rho }}_{0}]{\hat{j}}_{i}(0)\right\}\\ &=&\frac{1}{{{{\rm{i}}}}\hslash }{{{\rm{Tr}}}}\left\{{\hat{\rho }}_{0}[{\hat{D}}_{i^{\prime} }(0),{\hat{j}}_{i}(-t)]\right\}.\end{array}$$

(18)

Taking the Fourier transform of Eq. (15), we obtain

$${\tilde{j}}_{i}(\omega )=-{\tilde{\sigma }}_{i,1}(\omega ){\tilde{E}}_{\sin }(\omega )+{\tilde{\sigma }}_{i,2}(\omega ){\tilde{E}}_{\cos }(\omega )$$

(19)

with

$$\begin{array}{lll}{\tilde{\sigma }}_{i,i^{\prime} }(\omega )\,=\,\frac{\pi {r}_{0}^{2}M}{\hslash \omega }\int\nolimits_{0}^{\infty }{{{\rm{Tr}}}}\left\{{\hat{\rho }}_{0}[{\hat{j}}_{i^{\prime} }(0),{\hat{j}}_{i}(t)]\right\}{{{{\rm{e}}}}}^{{{{\rm{i}}}}(\omega +{{{\rm{i}}}}\eta )t}dt\\ {\tilde{E}}_{\sin }(\omega )\,=\,\int\nolimits_{-\infty }^{\infty }{E}_{0}\sin (\omega t){{{{\rm{e}}}}}^{{{{\rm{i}}}}(\omega +{{{\rm{i}}}}\eta )t}dt\\ {\tilde{E}}_{\cos }(\omega )\,=\,\int\nolimits_{-\infty }^{\infty }{E}_{0}\cos (\omega t){{{{\rm{e}}}}}^{{{{\rm{i}}}}(\omega +{{{\rm{i}}}}\eta )t}dt,\end{array}$$

(20)

where η is the phenomenological rate of relaxation and we have used ${\hat{j}}_{i}(0)=\frac{1}{\pi {r}_{0}^{2}M}{\left.\frac{\partial {\hat{D}}_{i}(t)}{\partial t}\right|}_{t = 0}$. The expression for ${\tilde{\alpha }}_{i,i^{\prime} }(\omega )$ is the Kubo formula for the dynamical conductivity. Optical selection rules can be established by transformation to the basis $\{\left|s,q,\kappa \right\rangle \}$. The position operator is written as^52,53

$${\hat{\overrightarrow{r}}}={r}_{0}\cos \psi {\overrightarrow{e}}_{x}+{r}_{0}\sin \psi {\overrightarrow{e}}_{y}+\left({{{\rm{i}}}}\sin \beta \frac{\partial }{\partial \kappa }+\sin \beta \hat{{{\Omega }}}\right){\overrightarrow{e}}_{z},$$

(21)

where β is the angle between the rotational and helical directions, and

$$\hat{{{\Omega }}}=\mathop{\sum}\limits_{s,s^{\prime} ,q,\kappa }{{{\rm{i}}}}\int {u}_{s^{\prime} ,q,\kappa }^{* }(\overrightarrow{r})\frac{\partial {u}_{s,q,\kappa }(\overrightarrow{r})}{\partial \kappa }{{{\rm{d}}}}\overrightarrow{r}\left|s^{\prime} ,q,\kappa \right\rangle \left\langle s,q,\kappa \right|$$

(22)

with ${u}_{s,q,\kappa }(\overrightarrow{r})$ being the Bloch function. We have

$${\hat{\rho }}_{0}=2\mathop{\sum}\limits_{s,q,\kappa }{n}_{F}({\varepsilon }_{s,q,\kappa })\left|s,q,\kappa \right\rangle \left\langle s,q,\kappa \right|$$

(23)

with n_F being the Fermi distribution. Substitution of Eqs. (21)–(23) into Eq. (20) yields the nonzero dynamical conductivity

$$\begin{array}{ll}{\tilde{\alpha }}_{1,1}(\omega )={\tilde{\alpha }}_{2,2}(\omega )\\ \qquad\quad\,\,=\dfrac{{{{\rm{i}}}}2{e}^{2}}{\pi {r}_{0}^{2}M{\hslash }^{2}\omega }\mathop{\sum}\limits_{s,s^{\prime} ,q^{\prime} ,\kappa }\left({n}_{F}({\varepsilon }_{s,q^{\prime} ,\kappa })-{n}_{F}({\varepsilon }_{s^{\prime} ,q^{\prime} -q,\kappa -{\kappa }_{0}})\right)\\ \displaystyle \qquad\quad\, \times \frac{| \left\langle s,q^{\prime} ,\kappa \right|[{\hat{H}}_{0},{r}_{0}]{{{{\rm{e}}}}}^{{{{\rm{i}}}}\psi }\left|s^{\prime} ,q^{\prime} -q,\kappa -{\kappa }_{0}\right\rangle {| }^{2}}{-| {\varepsilon }_{s^{\prime} ,q^{\prime} -q,\kappa -{\kappa }_{0}}-{\varepsilon }_{s,q^{\prime} ,\kappa }| +\hslash \omega +{{{\rm{i}}}}\hslash \eta },\end{array}$$

(24)

where we have used Eq. (16) and the rotating wave approximation. Therefore, ${\tilde{j}}_{1}(\omega )=-{\tilde{\sigma }}_{1,1}(\omega ){\tilde{E}}_{\sin }(\omega )$ and ${\tilde{j}}_{2}(\omega )={\tilde{\sigma }}_{2,2}(\omega ){\tilde{E}}_{\cos }(\omega )$. Equation (24) implies that transitions are allowed between bands with the OAM difference equal to the PAM, since in the numerator the OAM difference between the two states (q) is the OAM of the twisted light. In other words, the OAM is conserved modulo N. We show in Fig. 5a the band structure of the (5, 10) CNT with the OAM of the bands indicated. The transitions of an electron from the state $\left|-,-2,0\right\rangle$ to different conduction states are illustrated in Fig. 5b, showing that κ₀ depends on the PAM.

Fig. 5: Momentum conservation.

a Band structure of the (5,10) CNT. The bands are associated with OAM = −2 (red), −1 (green), 0 (black), +1 (blue), or +2 (orange). b Absorption of helical moment by an electron in state $\left|-,-2,0\right\rangle$ when illuminated by twisted light with different PAM (numbers at arrows).

Former studies of the optical selection rules by k ⋅ p theory⁵⁴ or, equivalently, by linear expansion of the Hamiltonian at the Dirac points of graphene⁵² did not consider the change of the symmetry group from SO(2) to C_N with the consequence that the OAM of both the twisted light and electron could take any integer value for all CNTs. This leads to wrong conclusions. For example, in the case of C₃ symmetry, electrons transfer from the valence band with q = 1 under illumination with l = 2 twisted light to the conduction band with q = 3 according to k ⋅ p theory, while they transfer to the conduction band with q = 0 according to Eq. (24).

Equation (5) has exactly the same form as the tight-binding model for an M-site chain of N-arm cofacial molecules⁴. Therefore $\mathop{\sum }\nolimits_{n = 1}^{N}{\varepsilon }_{N}^{(n-1)q}|{\phi }_{m,n}^{\alpha }\rangle$ can be considered as a twisted electronic state with OAM = q at site m. The two carbon atoms in one arm have the same phase terms, ${\varepsilon }_{M}^{(m-1)\kappa }$ and ${\varepsilon }_{N}^{(n-1)q}$, so that each CNT can be considered as a spiral of N-arm sites. In order for the exciton to carry nonzero OAM, we require N≥3, with N being the greatest common divisor of n₁ and n₂. This condition must be fulfilled in addition to the requirement that the CNT is semiconducting, see Eq. (4). From Eq. (7), one can expect that each band is associated with a specific OAM. This is demonstrated in Fig. 5, where the band structure of the (5,10) CNT is plotted with different colors encoding the OAM. The electrons and holes fulfill EAM = − HAM for a specific band.

OAM conservation between the photon of twisted light absorbed by the CNT and the twisted exciton (XAM = EAM + HAM) is confirmed by our analysis. For example, when an electron in the valence band with OAM = −1 absorbs twisted light with PAM = +2 it is excited into a conduction band with OAM = +1. Thus we have an electron with EAM = +1 and a hole with HAM = +1, such that the exciton carries XAM = EAM + HAM = PAM. In general, a twisted exciton with XAM = q + nN ($q\in [-\frac{N-1}{2},\frac{N-1}{2}]$) for arbitrary $n\in {\mathbb{Z}}$ is equivalent to the twisted exciton with XAM = q. This is analogous to the lack of uniqueness in the definition of crystal momentum, i.e., ℏk = ℏ(k + 2πn/a) where a is the lattice spacing. As a consequence, a twisted exciton with XAM = q can be generated by twisted light with PAM = q + nN. When N is even, excited states induced by twisted light with ∣q + nN∣ = N/2 have no specific circularity and therefore are not considered⁵⁵.

It is worth emphasizing the difference between the important role of the PAM here in contrast to the typically neglected role of the photonic linear momentum in standard band structure theory. In the latter case the linear momentum of the electrons in the valence band is so large that their change in momentum due to photon absorption can be safely ignored at room temperature. Within the CNT setting, though, the PAM is on the same order of magnitude as the EAM of the valence band electrons and the OAM conservation is nontrivial.

RT-TDDFT analysis

RT-TDDFT considers multi-level and multi-electron effects omitted in the tight-binding paradigm⁵⁶. And it is a computationally cheaper way to study excitonic effects comparing with the Bethe-Salpeter equation. The evolution of the electronic states during the interaction with twisted light can be explicitly tracked within a Kohn–Sham (KS) formulation of the electronic structure, see the Methods section for details. Exciton transport with conserved OAM is demonstrated for a 4 nm long (3, 3) CNT, see Fig. 6a, with hydrogenated ends, because a (5, 10) CNT with sufficient length exceeds the available computational resources. Note that the length of 4 nm is much larger than the diameter (~exciton size). The fact that the (3, 3) CNT is metallic does not prohibit demonstration of the core idea since it still has rotational and helical symmetries and has a small gap between highest occupied and lowest unoccupied orbitals for a finite length tube. A short laser pulse is applied to excite an exciton wave packet and a long laser pulse is applied to verify the OAM conservation, see Fig. 6c. The short laser pulse is localized in the time domain and delocalized in the frequency domain, see Fig. 6d, i.e., states in a broad range of energy are excited. In contrast, the long laser pulse is localized around 2 eV, which is less than the energy difference between the occupied and unoccupied KS orbitals with OAM = ±1, see Fig. 6b.

Fig. 6: The (3, 3) CNT.

a Decomposition of the (3, 3) CNT into a spiral of 3-arm sites. b Energies of the KS orbitals. The 402^nd is the highest occupied KS orbital. Orbitals with OAM = 0 and ±1 are shown in black and cyan, respectively. c Laser pulses with E₀ = 0.5 V/Å, ℏω = 2 eV, and envelope function $\exp (-{(t-0.3{{{\rm{fs}}}})}^{2}/2{(0.03{{{\rm{fs}}}})}^{2})$ (red) or $\exp (-{(t-10{{{\rm{fs}}}})}^{2}/2{(3{{{\rm{fs}}}})}^{2})$ (green). The gray curve is $0.5\sin (\omega t)$. d Normalized Fourier transforms of the curves in c.

Solution of the Bethe-Salpeter equation shows that strongly bound excitons dominate the excitations of the (3, 3) carbon nanotube⁵⁷. The absorption spectra without and with electron-hole interaction both feature a single prominent peak, which is found below the unbound electron-hole excitations in the case with electron-hole interaction due to the large exciton binding energy of ~100 meV. These results agree with the experimental observations of ref. ⁵⁸. The excitons could dissociated in an applied electric field⁵⁹ and in the internal electric field present at a p-n junction²⁶. In our case, however, there are no such fields. The excitons could also dissociate spontaneously, which is believed to be the reason for the observed photocurrent in carbon nanotubes despite the large exciton binding energy⁵⁹. The spontaneous dissociation is accompanied by phonon emission, as direct transition has been shown to be very weak⁶⁰. Since we do not consider phonons in our simulations, we can conclude that the excitations are excitons during and after the illumination.

To demonstrate the transport of an exciton wave packet along the CNT, the laser pulse is applied only to the first site of the CNT. The physics would not change when it was applied to more sites simultaneously, but this would require a longer CNT to observe the exciton wave packet. With phonon coupling disregarded, the laser pulse results in a coherent superposition of the ground and excited states. The electron and hole populations at each site are calculated through integration of the attachment and detachment densities⁶¹ over a cylinder with the site in the center and with the length being the projection of $\overrightarrow{H}$ on the axis of the CNT. The exciton population is defined as the average of the electron and hole populations. Figure 7 demonstrates that the electrons, holes, and excitons propagate along the CNT. Due to reflection at the other end, finally an equal distribution is established.

Fig. 7: Exciton wave packet.

a Electron and hole populations at different sites as functions of time. b Exciton population at different sites at specific times. The results refer to the short laser pulse of Fig. 6c.

To verify the OAM conservation, we first calculate the exciton populations when the system is initially in the ground state. The idea is that small excitations with different OAMs do not influence each other. Note that the long laser pulse in Fig. 6c is employed in the following. Let us denote the excited states with the same OAM (modulo N) by $\left|{{\Psi }}({{{\rm{OAM}}}})\right\rangle$ and the corresponding populations by p(OAM). If there is OAM conservation, then the excited state is $\left|{{\Psi }}(q)\right\rangle$ and its population is p(q) for twisted light with PAM = q. The total exciton population is ∑_qp(q) when light pulses with different q are applied. This is consistent with the observation p₄ ~ p₁ + p₃ and p₅ ~ p₁ + p₂ + p₃ in Fig. 8. When two pulses of the same twisted light are applied we have 4 times larger population, which is consistent with the observation p₆ ~ 4p₁ in Fig. 8. However, we yet cannot conclude that there is OAM conservation. If p₁ in Fig. 8 corresponds to an excited state $a\left|{{\Psi }}(1)\right\rangle +b\left|{{\Psi }}(-1)\right\rangle$, then p₃ must correspond to the excited state $b\left|{{\Psi }}(1)\right\rangle +a\left|{{\Psi }}(-1)\right\rangle$ and we have p₁ = p₃ = a² + b². Thus, the excited state induced by the two twisted light pulses is $(a+b)\left|{{\Psi }}(1)\right\rangle +(a+b)\left|{{\Psi }}(-1)\right\rangle$ and its population is p₄ = 2∣a + b∣². Assuming $a,b\in {\mathbb{R}}$, we find a² ~ 2400b² at t = 25 fs, which means that twisted light with a specific PAM induces an exciton with pure OAM.

Fig. 8: Twisted light to twisted exciton.

Exciton populations in a 4 nm long (3, 3) CNT illuminated by a twisted light pulse with PAM = −1 (p₁, p₇), 0 (p₂), or 1 (p₃, p₈), twisted light pulses with PAM = −1 and 1 of the same amplitude (p₄), twisted light pulses with PAM = −1, 0, and 1 of the same amplitude (p₅), and two twisted light pulses with PAM = −1 of the same amplitude (p₆). The CNT is initially in the ground state for p₁ to p₆ and in a 0.95:0.05 superposition of the ground state and excited states for p₇ and p₈.

Second, we calculate the exciton populations when the system is initially in an excited state with OAM = 1 and population 0.05 (p₇ and p₈ in Fig. 8). Specifically, one electron is initially excited from a linear combination of the 389^th and 390^th KS orbitals to the 403^rd KS orbital. Since we know that twisted light with PAM = ± 1 induces an exciton with pure OAM = ± 1, we will have either p₇ = 0.05 + p₁ or p₈ = 0.05 + p₃. Figure 9 shows p₇ ~ 0.05 + p₁, which means that the initial excited state has opposite OAM as compared to the excited state with p₁. In other words, the twisted light with PAM = − 1 induces an exciton with the same OAM, i.e., the OAM is conserved. Note that the initial excited state has an energy of 2.3 eV, which is not resonant with the frequency of the laser pulse. Thus, it contributes little to the excited state induced by the laser pulse and p₈ is similar to 0.05 + p₃.

Fig. 9: Exciton population differences.

p₇ − (0.05 + p₁) (green) and p₈ − (0.05 + p₃) (blue), compare Fig. 8. Black dashed lines indicate the average in the respective region.

Due to the OAM conservation, the population for each XAM can be determined, see the Methods section for details. Figure 10a shows $\overline{{{{\rm{XAM}}}}}$ for twisted light with PAM = −1, 0, and 1. We can conclude that twisted light with PAM = 0 leads to $\overline{{{{\rm{XAM}}}}}=0$ and twisted light with PAM = ± 1 leads to opposite $\overline{{{{\rm{XAM}}}}}$s. Using PAM = −1 as an example, we have $\overline{{{{\rm{XAM}}}}}=-p(-1)+2p(2)$. Together with the normalization condition p(−1) + p(2) = 1 this leads to the exciton populations shown in Fig. 10b. The population p(2) is due to excitations from occupied to unoccupied orbitals with OAM = ± 1, which have energies of at least 3 eV, see Fig. 6b, i.e., far from resonance with the frequency of the laser pulse. Therefore, p(2) can be neglected and the OAM can be determined in this case.

Fig. 10: OAM conservation.

a $\overline{{{{\rm{XAM}}}}}$ obtained for twisted light with PAM = −1 (green), 0 (black), and 1 (blue). b Corresponding exciton populations.

We next demonstrate conservation of the spin of circularly polarized light, which can be decomposed into radially and azimuthally polarized twisted lights^1,62,

$$\begin{array}{lll}\frac{1}{\sqrt{2}}({\overrightarrow{e}}_{x}\pm {{{\rm{i}}}}{\overrightarrow{e}}_{y})\\ =\frac{1}{\sqrt{2}}[(\cos \phi {\overrightarrow{e}}_{r}\mp \sin \phi {\overrightarrow{e}}_{\phi })\pm {{{\rm{i}}}}(\sin \phi {\overrightarrow{e}}_{r}\pm \cos \phi {\overrightarrow{e}}_{\phi })]\\ =\frac{1}{\sqrt{2}}{{{{\rm{e}}}}}^{\pm {{{\rm{i}}}}\phi }({\overrightarrow{e}}_{r}\pm {{{\rm{i}}}}{\overrightarrow{e}}_{\phi }),\end{array}$$

(25)

where ${\overrightarrow{e}}_{x}$, ${\overrightarrow{e}}_{y}$ and ${\overrightarrow{e}}_{r}$, ${\overrightarrow{e}}_{\phi }$ are the unit vectors of Cartesian and polar coordinate systems, respectively, and e^±iϕ represents OAM = ± 1. Right-hand circularly polarized light with spin = −1 is applied along the axis of the CNT. According to Fig. 11, p₁₀ ~ 4p₁ and p₁₁ ~ p₃ + p₉ confirm the equivalence of right-hand circularly polarized light with spin = −1 and twisted light with PAM = −1 in the sense that they induce an exciton with the same $\overline{{{{\rm{XAM}}}}}$. Thus, the CNT can convert circularly polarized light into twisted light.

Fig. 11: Circularly polarized light to twisted exciton.

Exciton populations in a 4 nm long (3, 3) CNT illuminated by right-hand circularly polarized light (p₉), right-hand circularly polarized light with spin = −1 and twisted light with PAM = −1 of the same amplitude (p₁₀), and right-hand circularly polarized light and twisted light with PAM = 1 of the same amplitude (p₁₁). The CNT is initially in the ground state. The p₁ = p₃ curve is reproduced from Fig. 8.

Discussion

We have shown that the orbital angular momentum is conserved during the excitation and subsequent transfer of twisted excitons in CNTs. These have extremely low reorganization energy in comparison to molecular chains implying the possibility of strong band transport and long coherence time for twisted exciton wave packets. CNTs therefore hold promise for exploiting the interplay of photonic and excitonic angular momentum for quantum information processing. The present work initially employs tight-binding analysis based on the helical and rotational symmetries to derive the dynamical conductivity. The obtained optical selection rules show OAM conservation and non-vertical transitions between bands for OAM ≠ 0. The advantage of using the helical and rotational symmetries is that the C_N rotation group of CNTs is considered automatically. Traditional derivations of the optical selection rules, by employing k ⋅ p theory or equivalently by linear expansion of the Hamiltonian around the Dirac points of graphene, are actually based on the rotation group SO(2), which leads to wrong conclusions on the optical excitations of CNTs illuminated by twisted light.

We employ the RT-TDDFT approach, which includes multi-level and multi-electron effects, being computationally cheaper than the Bethe-Salpeter equation, for the study of excitons. The results confirm that the OAM is conserved in the presence of electron-hole interaction, between photon and exciton as well as during exciton transfer. Former studies have shown that OAM cannot be exchanged between twisted light and the electrons of isolated atoms by dipole transition^{63,64,65,66,67}. However, this does not apply to a ring of atoms with C_N symmetry¹, as each atom can be excited by dipole transition and pick up the specific phase of the twisted light at its position, giving rise to a collective mode. The reverse process, generation of twisted light via the dipole transition of molecules in a ring, has been demonstrated in ref. ⁵⁵.

Methods

Time-dependent Kohn–Sham formulation

Denoting the external potential (nuclei and light field) as ν_ext, the Hartree potential as ν_Ha, and the exchange-correlation potential as ν_xc, we have

$$\begin{array}{ll}{{{\rm{i}}}}\hslash \frac{\partial }{\partial t}\left|{\psi }_{i}(r,t)\right\rangle =\left[-\frac{{\hslash }^{2}}{2m}{\nabla }^{2}+{\nu }_{ext}(r,t)+{\nu }_{Ha}[\rho ](r,t)\right.\\ \qquad\qquad\qquad\,\left.+\,{\nu }_{xc}[\rho ](r,t)\right]\left|{\psi }_{i}(r,t)\right\rangle \end{array}$$

(26)

$$\rho (r,t)=2\mathop{\sum }\limits_{i=1}^{N}\left|{\psi }_{i}(r,t)\right\rangle \left\langle {\psi }_{i}(r,t)\right|.$$

(27)

Eq. (27) is the spin-reduced density operator of 2N electrons. RT-TDDFT simulations are carried out using the Octopus package⁶⁸ with Troullier-Martins pseudopotentials and the Perdew–Burke–Ernzerhof exchange-correlation potential within the generalized gradient approximation. A time step of 0.66 × 10⁻¹⁸ s is employed together with an atomic sphere radius of 3 Å and a grid size of 0.15 Å.

Average OAM of the exciton

In principle, the total OAM can be calculated as the sum of the OAMs of all states weighted by their populations, which is, however, computationally impossible. The total OAM can also be calculated by summation of the OAMs over all occupied time-propagated KS orbitals³⁷. Because of the C_N symmetry, the KS orbitals with OAM = 0 are nondegenerate and the KS orbitals with OAM ≠ 0 are twofold degenerate. As we can always construct KS orbitals $\left|{\psi }_{j}^{q}(r,0)\right\rangle$ with OAM = q from $\left|{\psi }_{j}(r,0)\right\rangle$ by the method of ref. ¹, the OAM of the i-th time-propagated KS orbital can be readily calculated as

$${{{{\rm{OAM}}}}}_{i}=\mathop{\sum }\limits_{j=1}^{M}| \langle {\psi }_{j}^{q}(r,0)| {\psi }_{i}(r,t)\rangle {| }^{2}q.$$

(28)

Thus, $\mathop{\sum }\nolimits_{i = 1}^{N}{{{{\rm{OAM}}}}}_{i}$ is the total OAM of the time-propagated multi-electron state, which is a linear combination of the ground and excited states. Since the ground state has OAM = 0, the average OAM of the exciton is

$$\overline{{{{\rm{XAM}}}}}=\mathop{\sum }\limits_{i=1}^{N}{{{{\rm{OAM}}}}}_{i}/\mathop{\sum }\limits_{j=1}^{M/2}{n}_{j}^{2},$$

(29)

with the total number $\mathop{\sum }\nolimits_{j = 1}^{M/2}{n}_{j}^{2}$ of excitons derived from the positive eigenvalues {n_j} of (ρ(r, t) − ρ(r, 0))/2⁶¹. The overline indicates that this is an average quantity.

Eq. (29) does not recognize the equivalence of OAMs modulo N³⁷. For example, in the case of the (3, 3) CNT we have

$$\overline{{{{\rm{XAM}}}}}=\mathop{\sum }\limits_{q,q^{\prime} =-1}^{1}{p}_{q^{\prime} }^{q}(q-q^{\prime} ),$$

(30)

where ${p}_{q^{\prime} }^{q}$ is the population of the state with one electron excited from the KS orbital with OAM $=q^{\prime}$ to that with OAM = q (the total population of the excited states being normalized to 1). When twisted light with PAM = 1 is applied, we have $\overline{{{{\rm{XAM}}}}}={p}_{0}^{1}(1)+{p}_{1}^{-1}(-2)$, which is not equal to the PAM when ${p}_{1}^{-1}\ne 0$. While Eq. (29) thus cannot be used to determine the total OAM of the excitons, it helps to determine the population of each exciton with distinct OAM.